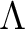Next: sheaves Up: Zariski topology on affine Previous: compactness
 be a commutative ring. Let
be a commutative ring. Let  be a
subset of
be a
subset of  . Then we define
. Then we define 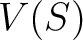 as follows.
as follows.
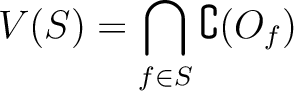
 .
.
 be a commutative ring.
Then for any subset
be a commutative ring.
Then for any subset  of
of  , we have the following.
, we have the following.
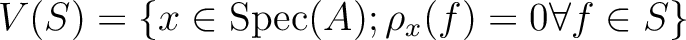
 where
where  is the ideal of
is the ideal of  generated by
generated by  .
.


Thus a closed set in
 is of the form
is of the form  for some ideal
for some ideal 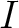 .
.
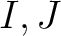 of a commutative ring
of a commutative ring  , we have the following.
, we have the following.
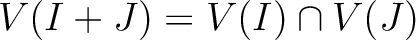 .
.
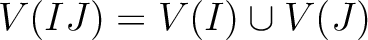 .
.
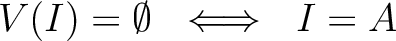 .
.
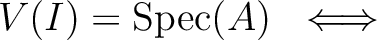 any element of $I$ is nilpotent.
any element of $I$ is nilpotent.
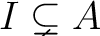 , then by the Zorn's lemma we obtain a maximal ideal
, then by the Zorn's lemma we obtain a maximal ideal
 which contains
which contains 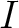 . Since maximal ideals are prime,
we have
. Since maximal ideals are prime,
we have
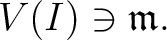
 is not empty. The converse is obvious.
is not empty. The converse is obvious.
(4)is a consequence of Lemma 7.11.
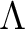
The reader may easily see that the compactness of
 (Theorem 7.12) is proved in a more easier way
if we have used the terms of closed sets and “finite intersection property”.
(Theorem 7.12) is proved in a more easier way
if we have used the terms of closed sets and “finite intersection property”.
The author cannot help but mentioning little more
how the topology of
 and
the structure of
and
the structure of  related to each other.
related to each other.
Though the following statements may never be used in this talk (at least in the near future), we would like to record the statement and its proof.
 be a ring.
be a ring.
 is not connected so that it is divided into
two disjoint closed sets
is not connected so that it is divided into
two disjoint closed sets  and
and 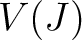 .
.

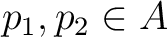 such that
such that
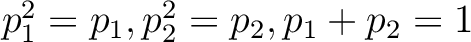
 is a product of algebras
is a product of algebras  ,
, 
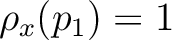 for all
for all 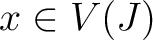 .
.
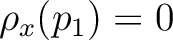 for all
for all  .
.
 has elements
has elements 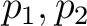 which satisfy (a)-(b) above,
then
which satisfy (a)-(b) above,
then
 is divided into two disjoint closed sets.
is divided into two disjoint closed sets.
 and
and 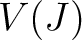 is disjoint, we have
is disjoint, we have
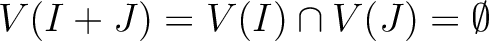
 . It follows that there exists
. It follows that there exists 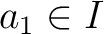 and
and  such that
such that  .
On the other hand,
.
On the other hand,
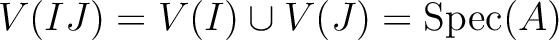
 is nilpotent.
Let
is nilpotent.
Let 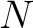 be a positive integer such that
be a positive integer such that
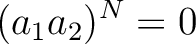

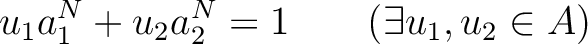
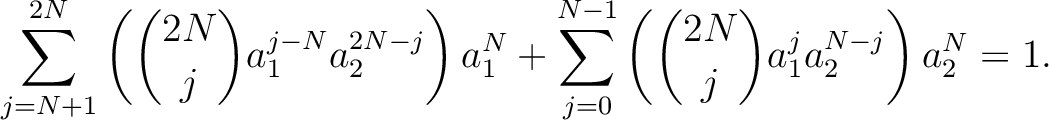
Now let us put
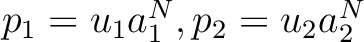 .
They satisfy
.
They satisfy
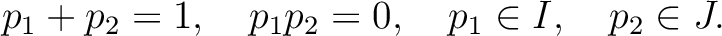
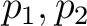 satisfy the
required properties.
The converse is easier and is left to the reader.
satisfy the
required properties.
The converse is easier and is left to the reader.