Resolutions of singularities.

Yoshifumi Tsuchimoto

From the paper of Encinas and Hauser:

&dotfill#dotfill;

The coefficient ideal of an ideal $I$ of $W$ at a with respect to $V$ is an ideal in $V$ which is built from the coefficients of the Taylor expansion of the elements of $I$ with respect to the equations defining $V$ . Let $x, y$ and $y$ be regular systems of parameters of $\mathcal O_{W,a}$ and $\mathcal O_{V,a}$ so that $x = 0$ defines $V$ in $W$ . For $f$ in $I$ denote by $a_{f,\alpha}$ the elements of $\mathcal O_{V,a}$ so that $f = \sum_\alpha a_{f,\alpha}\cdot x^\alpha$ holds after passage to the completion. Then we set

$$\operatorname{coeff}_V I = \sum_{\vert\alpha\vert<c} (a_{f,\alpha}; f\in I)^{\frac{c!}{c-\vert\alpha\vert}}.$$

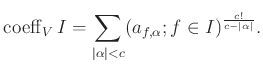
&dotfill#dotfill;

EXAMPLE 08.1   Let us consider a curve $C=\{(t^3,t^5,t^7)\}\in \mathbb{A}^3$ . By using a theory of groebner basis, we may eliminate the variable $t$ and obtain an ideal

$$I=(y^7-z^5,xz-y^2,xy^5-z^4,x^2y^3-z^3,x^3y-z^2,x^4-zy)$$

Let us choose $V=\{z=0\}$ as the hypersurface. Then:

1. When $c=1$ , we have
   $$\operatorname{coeff}_V(I)=(y^2,x^3y,x^4)$$

2. When $c=2$ , we have
   $$\operatorname{coeff}_V(I)=(y^2,x^3y,x^4)+(x,y)^2=(x^2,xy,y^2)$$

3. When $c=3$ , we have
   $$\operatorname{coeff}_V(I)=(y^2,x^3y,x^4)^2+(x,y)^3+(1)^6=(1).$$

PROBLEM 08.2   To compute the coefficient ideal of a given ideal $I$ , Is it sufficient to consider only coefficients of generators of $I$ ?

Let $X$ be a closed subscheme of a regular scheme $W$ . We want to resolve the singularity of $X$ . If there exists an regular hypersurface $V$ such that $V\supset X$ , then we may replace $W$ by $V$ . So we may (have to) assume that $X$ is not contained in such hypersurfaces. Instead, we have for each point $a\in X$ a ``hypersurface of maximal contact'' $V$ . $V$ is not canonical, but is good enough to define the invariant $i_a$ and then (afterwards) determine the center of blow up.