Categories, abelian categories and cohomologies.

Yoshifumi Tsuchimoto

DEFINITION 10.1   Let $A$ be a commutative ring. Let $S$ be its subset. We say that $S$ is multiplicative if

1. $1\in S$
2. $x,y \in S \implies x y \in S$

holds.

DEFINITION 10.2   Let $S$ be a multiplicative subset of a commutative ring $A$ . Then we define $A[S^{-1}]$ as

$$A[\{X_s ; s \in S\}]/(\{ s X_s -1; s \in S\})$$

where in the above notation $X_s$ is a indeterminate prepared for each element $s \in S$ .) We denote by $\iota_S$ a canonical map $A\to A[S^{-1}]$ .

LEMMA 10.3   Let $S$ be a multiplicative subset of a commutative ring $A$ . Then the ring $B=A[S^{-1}]$ is characterized by the following property:

Let $C$ be a ring, $\varphi:A\to C$ be a ring homomorphism such that $\varphi(s)$ is invertible in $C$ for any $s \in S$ . Then there exists a unique ring homomorphism $\psi=\phi[S^{-1}]:B\to C$ such that

$$\varphi=\psi \circ \iota_S$$

holds.

COROLLARY 10.4   Let $S$ be a multiplicative subset of a commutative ring $A$ . Let $I$ be an ideal of $A$ given by

$$I=\{ x \in I; \exists s \in S$$    such that $$s x=0\}$$

Then (1) $I$ is an ideal of $A$ . Let us put $\bar{A}=A/I$ , $\pi:A\to \bar{A}$ the canonical projection. Then:

(2) $\bar{S}=\pi(S)$ is multiplicatively closed.

(3) We have

$$A[S^{-1}]\cong\bar{A}[\bar{S}^{-1}]$$

(4) $\iota_{\bar{S}}: \bar{A}\to \bar{A}[\bar{S}^{-1}]$ is injective.

DEFINITION 10.5   Let $S$ be a multiplicative subset of a commutative ring $A$ . Let $M$ be an $A$ -module we may define $S^{-1}M$ as

$$\{ (m/s); m\in M , s\in S\} / \sim$$

where the equivalence relation $\sim$ is defined by

$$(m_1/s_1)\sim (m_2/s_2) \iff t (m_1 s_2 -m_2 s_1)=0 \quad (\exists t \in S).$$

We may introduce a $S^{-1}A$ -module structure on $S^{-1}M$ in an obvious manner.

$S^{-1}M$ thus constructed satisfies an universality condition which the reader may easily guess.

LEMMA 10.6   Let $A$ be a commutative ring. Let $M$ be an $A$ -module. Then we have a canonical isomorphism of $A_S$ module

$$A_S \otimes_A M \cong M_S.$$

We may also localize categories, but we need to deal with non commutativity of composition. To simplify the situation we only deal with a localization with some nice properties as follows:

1. 1. $s, t \in \Sigma \implies s t \in \Sigma$
   2. $X\in \operatorname{Ob}(\mathcal{C})\implies 1_X \in \Sigma$ .
2. Let $X,Y,Z\in \operatorname{Ob}(\mathcal{C})$ . Let $u\in \operatorname{Hom}_\mathcal{C}(X,Y)$ , $s\in \operatorname{Hom}_\mathcal{C}(Z,Y)\cap \Sigma$ . Then there exist $W\in \operatorname{Ob}(\mathcal{C})$ and morphisms $v\in \operatorname{Hom}_\mathcal{C}(W,Z)$ , and $t\in \operatorname{Hom}_\mathcal{C}(W,X)\cap \Sigma$ such that the diagram
   $$\begin{CD} W @>v» Z \\ @Vt VV @V sVV \\ X @> u» Y \end{CD}$$
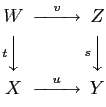
   commutes.

   In a simpler (but not rigorous) words, for each ``composable $s^{-1} u$ '', there exists $v,t$ such $s^{-1}u= v t^{-1}$ . Similarly, for each composable $u s^{-1}$ , there exists $v,t$ such that $u s^{-1}=t^{-1} v$ holds.

3. Let $X,Y\in \operatorname{Ob}(\mathcal{C})$ , $u,v\in Hom_\mathcal{C}(X,Y)$ . Then the following conditions are equivalent:
   1. There exists $Y'\in \operatorname{Ob}(\mathcal{C})$ and $s \in \operatorname{Hom}_\mathcal{C}(Y,Y')\cap \Sigma$ such that $su=sv$ .
   2. There exists $X'\in \operatorname{Ob}(\mathcal{C})$ and $t \in \operatorname{Hom}_\mathcal{C}(Y,Y')\cap \Sigma$ such that $u t =v t$ .
4. If $s \in \Sigma$ and if $su\in \Sigma$ then $u \in \Sigma$ .

LEMMA 10.7   Let $\Sigma$ be a family of morphisms in $\mathcal{C}$ which satisfies the properties above. Then one may construct a localization of $\mathcal{C}_\Sigma$ with respect to $\Sigma$ . Furthermore, if $\mathcal{C}$ is additive, then $\mathcal{C}_\Sigma$ is also additive.