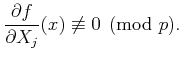Next: Bibliography
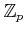 ,
,
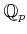 , and the ring of Witt vectors
, and the ring of Witt vectors
No.06:

DEFINITION 06.1
We denote by
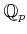
the quotient field of
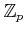
.
LEMMA 06.2
Every non zero element
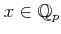 is uniquely expressed as
is uniquely expressed as
We have so far constructed a ring
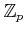 and a field
and a field
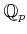 for each prime
for each prime 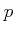 .
.
With
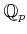 and/or
and/or
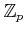 , we may do some ``calculus'' such as:
, we may do some ``calculus'' such as:
THEOREM 06.4
[1, corollary 1 of theorem 1]
Let
![$ f\in \mathbb{Z}_p[X_1,X_2,\dots,X_m], x\in \mathbb{Z}_p^m$](img9.png) ,
,
 .
Assume that there exists a natural number
.
Assume that there exists a natural number  such that
such that
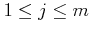 ,
,
Then there exists
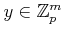 such that
such that
|
(1) |
|
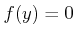 |
|
(2) |
|
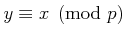 |
See [1] for details.



Next: Bibliography
2008-06-10
![]() and a field
and a field
![]() for each prime
for each prime ![]() .
.
![]() and/or
and/or
![]() , we may do some ``calculus'' such as:
, we may do some ``calculus'' such as: