


: この文書について...
代数学 I No.8要約
《割り算の原理(ユークリッド環)》
これ以降、この講義では「環」と単に言えば可換環のことを
指すことにする。
 と
と ![$ k[X]$](img3.png) の二つにまず共通して言えることは、
どちらも「余りのある割り算」が出来ることである。
の二つにまず共通して言えることは、
どちらも「余りのある割り算」が出来ることである。
余りのある割り算なら出来るのが当たり前のことに思えるかも知れない。しかし、
たとえば
![$ {\mbox{${\mathbb{Z}}$}}[X]$](img4.png) の中で考えて
の中で考えて 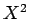 を
を 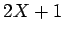 で割った余りは?
で割った余りは?
![$ {\mathbb{C}}[X,Y]$](img7.png) の中で考えて
の中で考えて 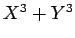 を
を  で割った余りは?
で割った余りは?
などと聞かれると困ってしまう。
ポイントは、
「どこで割り算が終ったか分かるような尺度があるかどうか」
という点にある。そこで次のような定義をする。
(「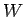 が整列集合である」とは、
が整列集合である」とは、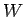 は順序集合であって、しかも
「
は順序集合であって、しかも
「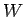 の任意の部分集合
の任意の部分集合 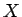 は最小元を持つ」というときにいう。
この定義が難しく感じられる諸君には
は最小元を持つ」というときにいう。
この定義が難しく感じられる諸君には
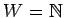 と思っても実用上は充分である。
)
と思っても実用上は充分である。
)
補題 8.1 (ユークリッド環の基本例)

,
![$ k[X]$](img3.png)
(
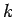
は体)はともにユークリッド環である。
割り算の原理としては次のこともよく使う。
補題 8.2 (モニックな多項式による割り算)
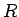
を単位元を持つ可換環とする。
![$ R[X]$](img24.png)
の元
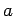
がモニックならば、
任意の
![$ b\in R[X]$](img25.png)
に対して、
となる
![% latex2html id marker 1032
$ q,r\in R[X]$](img27.png)
が存在する。
定義 8.2
環
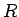
のイデアル
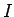
が単項イデアルであるとは、
ある
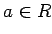
が存在して、
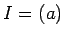
が成り立つときに言う。
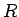 の全てのイデアルが単項イデアルであるとき、
の全てのイデアルが単項イデアルであるとき、
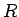 は単項イデアル環であると言う。
は単項イデアル環であると言う。
定理 8.1
ユークリッド環は単項イデアル環である。
系 8.1
整数
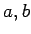
が与えられているとし、その最小公約数を
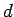
とおく。このとき、
をみたす整数
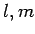
が存在する。
系 8.2
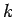
を体とする。
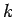
上の多項式
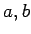
が与えられているとし、その最小公約数を
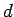
とおく。このとき、
をみたす
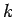
上の多項式
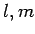
が存在する。
実際に 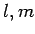 を計算するには、次のような方法が便利である。
を計算するには、次のような方法が便利である。
例題 8.1 (ユークリッドの互除法)
等式
を満たす整数
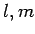
の組を一組求めよ。
(解答)
まず次のような計算を行なう
各々の行の行列算を組み合わせると、
を得る。この式の右辺に現れる正方行列はすべて
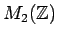 の元として
可逆であることに注意して、上の式を次のように変形することが出来る。
の元として
可逆であることに注意して、上の式を次のように変形することが出来る。
この式の第一行に着目すると、
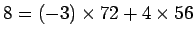 を得る。
を得る。
(答え)
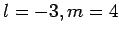 .
.
次の補題は単項イデアルの包含関係を生成元の関係に翻訳しなおしている。
※レポート問題
つぎのうち一問を選択して解きなさい。
(期限:次の講義の終了時まで。)
- (I).
- 等式
を満たす多項式
![$ f,g\in {\mathbb{C}}[X]$](img68.png) の組を一組見つけなさい。
今回はその見付けかたまで込めて書くこと。
の組を一組見つけなさい。
今回はその見付けかたまで込めて書くこと。
- (II).
-
![% latex2html id marker 1138
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{-1}]$](img69.png) はユークリッド環である。
(その証明は本問題では書かなくてもよいことにする。
はユークリッド環である。
(その証明は本問題では書かなくてもよいことにする。
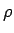 としては《絶対値》を考え、
としては《絶対値》を考え、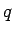 としては
としては 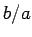 にもっとも近い
にもっとも近い
![% latex2html id marker 1146
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{-1}]$](img73.png) の元をとればよい。
(
の元をとればよい。
(
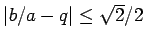 に出来る。))
このことを用いて、
に出来る。))
このことを用いて、
![% latex2html id marker 1150
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{-1}]$](img75.png) の元
の最大公約数をユークリッドの互除法を用いて求めなさい。
の元
の最大公約数をユークリッドの互除法を用いて求めなさい。



: この文書について...
平成19年11月29日
![]()
![]() と
と ![]() の二つにまず共通して言えることは、
どちらも「余りのある割り算」が出来ることである。
の二つにまず共通して言えることは、
どちらも「余りのある割り算」が出来ることである。
![]() の中で考えて
の中で考えて ![]() を
を ![]() で割った余りは?
で割った余りは?
![]() の中で考えて
の中で考えて ![]() を
を ![]() で割った余りは?
で割った余りは?
![]() の全てのイデアルが単項イデアルであるとき、
の全てのイデアルが単項イデアルであるとき、
![]() は単項イデアル環であると言う。
は単項イデアル環であると言う。
![]() を計算するには、次のような方法が便利である。
を計算するには、次のような方法が便利である。
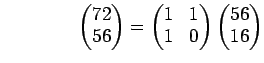
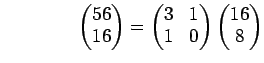
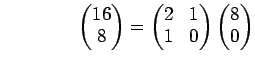


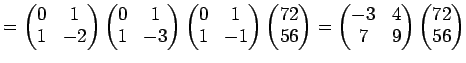
![]() .
.