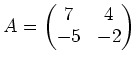: この文書について...
代数学 A No.7要約
 《環の準同型定理の利用法》
《環の準同型定理の利用法》
例 7.1 (準同型定理の基本例1)
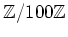
から
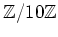
への写像
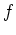
を、
![% latex2html id marker 1002
$\displaystyle f([n]_{100})=[n]_{10} \quad \quad ($](img5.png)
$[?]_n$ は $$

$/n$

$$ における
$?$ の同値類
で定めると、次のことが分かる。
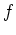 は写像としてうまく定義されている。
すなわち、
は写像としてうまく定義されている。
すなわち、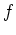 の定義は代表元のとり方によらない。
の定義は代表元のとり方によらない。
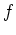 は環の準同型である。
は環の準同型である。
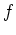 の像は
の像は
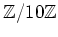 全体である。
全体である。
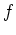 の核は
の核は
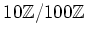 である。
である。
よって、準同型定理により、
が結論される。
例 7.2 (準同型定理の応用例1)
![$ {\mbox{${\mathbb{Z}}$}}[X]$](img10.png)
から
![% latex2html id marker 1026
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img11.png)
への写像
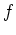
を、
で定めると、次のことが分かる。
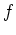 は写像としてうまく定義されている。
すなわち、
は写像としてうまく定義されている。
すなわち、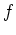 の像は
の像は
![% latex2html id marker 1036
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img13.png) からはみ出さない。
からはみ出さない。
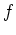 は環の準同型である。
は環の準同型である。
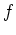 の像は
の像は
![% latex2html id marker 1042
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img14.png) 全体である。
全体である。
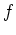 の核は
の核は
![% latex2html id marker 1046
$ (X^2-14){\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img15.png) である。
である。
よって、準同型定理により、
が結論される。
例 7.3 (準同型定理の応用例2)
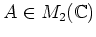
を、
で定め、
![$ {\mathbb{C}}[X]$](img19.png)
から
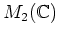
への写像
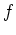
を、
で定めると、次のことが分かる。
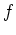 は環の準同型である。
は環の準同型である。
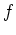 の像は
である。
の像は
である。
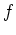 の核は
の核は
![$ (X^2-8 X-8){\mathbb{C}}[X]$](img23.png) である。
である。
よって、準同型定理により、
が結論される。
※レポート問題
つぎのうち一問を選択して解きなさい。
(期限:次の講義の終了時まで。)
- (I).
- 環としての同型
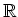
![$ [X]/(X^2+1)$](img26.png)
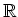
![$ [X] \cong {\mathbb{C}}$](img27.png) が
存在することを示しなさい。
が
存在することを示しなさい。
- (II).
-
と置くとき、
-
![$ {\mathbb{C}}[A] \cong {\mathbb{C}}[X]/(X^2-5X+6){\mathbb{C}}[X]$](img29.png) であることを示しなさい。
であることを示しなさい。
-
![$ {\mathbb{C}}[X]/(X^2-5X+6){\mathbb{C}}[X]$](img30.png) の 0 でない零因子を一つあげなさい。
の 0 でない零因子を一つあげなさい。
-
![$ {\mathbb{C}}[A]$](img31.png) の 0 でない零因子を一つあげなさい。
の 0 でない零因子を一つあげなさい。
平成17年11月24日
![]() 《環の準同型定理の利用法》
《環の準同型定理の利用法》
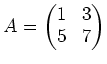
![$\displaystyle {\mathbb{C}}[A]=
{\mathbb{C}}A+{\mathbb{C}}E= \{k A+ lE ; k,l \in...
...in{pmatrix}
k+l &3k \\
5k &7k+l
\end{pmatrix}; k,l \in {\mathbb{C}}
\right \}
$](img22.png)