DEFINITION 10.1
Let

,
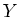
be schemes, and let
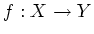
be a morphism. We say that
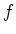
is of
finite type
if there exists an open cover

of
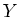
by affine schemes
and a finite open cover
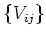
of each

by affine schemes
such that
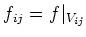
is ``a morphism of finite type''
for every
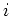
and
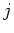
. That means, if we put
Then
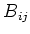
is finitely generated algebra over
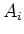
.